Boats and Streams Problems with Solutions for SSC and Bank Exams

Every student wants to solve all problems of boats and streams within less time in competitive exams but they can't solve questions on time. The reason behind it, students don't have practiced the sufficient examples to solve boats and streams problems.
So here in this article, you can learn the boats and streams problems with solutions for SSC and bank exams. Here are the examples also which will help you to solve boats and streams problems. You can practice boats and streams questions and answers.
Boats and Streams Problems with Solutions for Competitive Exams
Q.1. A man can row upstream at 8 kmph and downstream at 13 kmph. The speed of the stream is :
Solution:
$$ Speed \ of \ stream \ = {1\over2}(13-8)\ kmph=2.5 \ kmph$$
Q.2. A boat running downstream covers a distance of 16 km in 2 hours while for covering the same distance upstream, it takes 4 hours. What is the speed of the boat in still water?
Solution:
$$ Rate \ downstream =\left({16\over2}\right)kmph,$$
$$ Rate \ upstream =\left({16\over4}\right)kmph=4 \ kmph.$$
$$∴ Speed \ in \ still \ water={1\over2}(8+4)kmph=6kmph$$
Q.3. A man can row three-quarters of a kilometre against the stream in 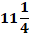 minutes. The speed (in km/hr) of the man in still water is
minutes. The speed (in km/hr) of the man in still water is
$$ Rate \ upstream =\left({750\over675}\right)m / sec={10\over9}m / sec$$
$$ Rate \ downstream =\left({750\over450}\right)=\left({5\over3}\right)m / sec$$
$$Rate\ in \ still\ water={1\over2}\left({10\over9}+{5\over3}\right)m / sec$$
$$={25\over18}m / sec=\left({25\over18}×{5\over3}\right)km/hr=5km/hr$$
Q.4. A man’s speed with the current is 15 km/hr and the speed of the current is 2.5 km/hr. The man’s speed against the current is
Solution:
Man’s rate in still water = (15 – 2.5) km/hr = 12.5 km / hr.
Man’s rate against the current = (12.5 – 2.5) km/hr = 10 km/hr.
Q.5. A boat can travel with a speed of 13 km/hr in still water. If the speed of the stream is 4 km/hr, find the time taken by the boat to go 68 km downstream.
Speed downstream = ( 13+4) km/ hr = 17 km / hr.
Time taken to travel 68 km downstream = 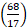 hrs=4hrs.
hrs=4hrs.
Q.6. In one hour, a boat goes 11 km along the stream and 5 km against the stream. The speed of the boat in still water (in km/hr) is :
Solution:
$$ Speed \ in \ still \ water = {1\over2}(11+5)\ kmph=8 \ kmph$$
Q.7. A man rows downstream 32 km and 14 km upstream. If he takes 6 hours to cover each distance, then the velocity (in kmph) of the current is :
Solution:
$$ Rate \ downstream =\left({32\over6}\right)kmph,$$
$$ Rate \ upstream =\left({14\over6}\right)kmph.$$
$$∴ Velocity \ of \ current={1\over2}\left({32\over6}-{14\over6}\right)kmph$$
$$={3\over2}kmph=1.5kmph$$
Q.8. A boatman goes 2 km against the current of the stream in 1 hour and goes 1 km along the current in 10 minutes. How long will it take to go 5 km in stationary water ?
Solution:
$$ Rate \ downstream =\left({1\over10}×60\right)km/hr=6km/hr;$$
$$ Rate \ upstream =2km/hr.$$
$$ Speed \ in \ still \ water = {1\over2}(6+2)\ km/hr =4 \ km/hr$$
$$∴ Required \ time =\left({5\over4}\right)hrs =1{1\over4}=1 \ hr \ 15 \ min $$
Q.9. If a boat goes 7 km upstream in 42 minutes and the speed of the stream is 3 kmph, then the speed of the boat in still water is :
Solution:
$$ Rate \ upstream =\left({7\over42}×60\right)kmph=10 \ kmph.$$
Speed of stream = 3kmph.
Let speed in still water be x km/hr. Then, speed upstream = (x-3) km/hr.
∴ x – 3 = 10 or x = 13 km/hr.
Q.10. If a man rows at the rate of 5 kmph in still water and his rate against the current is 3.5 kmph, then the man’s rate along the current is :
Let the rate along the current be x kmph.
$$Then, {1\over2}\left ({x+3.5}\right)=5 \ or \ x = 6.5 \ kmph$$
I hope these solutions were helpful for you or your upcoming exams. You can learn how to use formulas of boats and streams if you have any confusion. Ask me in the comment section if you have any doubt regarding boats and streams problems with solutions for ssc and bank exams.



