Number System Questions and Answers with Solution

In a division problem, the divisor is 4 times the quotient and 3 times the remainder. If reminder is 4, the dividend is :
(A) 36
(B) 40
(C) 12
(D) 30
Correct Answer : B
Explanation :
Remainder = 4
⇒ Divisor = 3 × 4 = 12
Again, divisor = 4 × quotient
⇒ 4 × quotient = 12
Quotient =
⇒ Dividend = 3 × 12 + 4 = 40
Each member of a Picnic party contributed twice as many rupees as the total collection was Rs.3042. The number of members present in the party was
(A) 2
(B) 32
(C) 42
(D) 39
Correct Answer : D
Explanation :
Let the required number of persons be x.
According to the question, 2x2 = 3042
Or 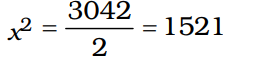
or 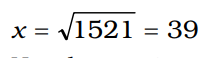
How many natural numbers divisible by 7 are there between 3 and 200?
(A) 28
(B) 29
(C) 27
(D) 36
Correct Answer : A
Explanation :
Number just greater than 3 which is divisible by 7 = 7
Number just smaller than 200 which is divisible by 7 = 196
Here, a = 7, an = 196,
d = 7, n = 8
an = a + (n –1)d
⇒196 = 7 + (n – 1) × 7
⇒ 
⇒ n = 27 + 1 = 28
Note : We can find the answer after dividing 200 by 7. The quotient is our answer.
The sum of first sixty numbers from one to sixty is divisible by
(A) 13
(B) 60
(C) 61
(D) 59
Correct Answer : C
Explanation :
Sum of first 60 numbers

The number 1830 is divisible by 61.
A number when divided by 3 leaves a remainder 1. When the quotient is divided by 2, it leaves a remainder 1. What will be the remainder when the number is divided by 6?
(A) 5
(B) 2
(C) 3
(D) 4
Correct Answer : D
Explanation :
The least number (dividend) x is obtained as follows :
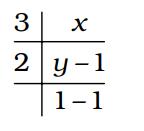
y = 2 × 1 + 1 = 3
x = 3 × 3 + 1 = 10
When we divide 10 by 6, the remainder = 4
The product of two numbers is 9375 and the quotient, when the larger one is divided by the smaller, is 15. The sum of the number is:
(A) 395
(B) 380
(C) 400
(D) 425
Correct Answer : C
Explanation :
Let the numbers be x and y and x is greater than y.
As given,
xy = 9375 ....(i)
Again,
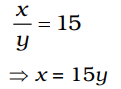
∴ From equation (i),
15y × y = 9375
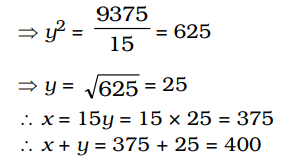
A number when divided by 119, leaves a remainder of 19. If it is divided by 17, it will leave a remainder of:
(A) 19
(B) 7
(C) 10
(D) 2
Correct Answer : D
Explanation :
On dividing the given number by 119, let k be the quotient and 19 as remainder.
Then, number = 119k + 19
= 17 × 7k + 17 × 1 + 2
= 17 (7k + 1) + 2
Hence, the given number when divided by 17, gives (7k + 1) as quotient and 2 as remainder.
(719 + 2) is divided by 6, the remainder is :
(A) 2
(B) 1
(C) 5
(D) 3
Correct Answer : D
Explanation :
By the Binomial expansion we have

Here, each term except the last term contains x. Obviously, each term except the last term is exactly divisible by x.
Following the same logic,
719 = (6 + 1)19 has each term except last term divisible by 6.
Hence, 719 + 2 when divided by 6 leaves remainder =1 + 2 = 3
When a number is divided by 357 the remainder is 39. If that number is divided by 17, the remainder will be:
(A) 5
(B) 11
(C) 0
(D) 3
Correct Answer : A
Explanation :
Here, 357 is exactly divisible by 17.
∴ Required remainder = Remainder obtained on dividing 39 by 17 = 5
A number divided by 68 gives the quotient 269 and remainder zero. If the same number is divided by 67, the remainder is:
(A) 2
(B) 3
(C) 0
(D) 1
Correct Answer : D
Explanation :
Number = 269 × 68
= 269 × (67 + 1)
= 269 × 67 + 269
Clearly, remainder is obtained on dividing 269 by 67 that is 1.



