Cube Questions Practice Question and Answer
8 Q: How many cubes are there which two adjacent sides are coloured with red or with black colour ?
1194 15f5898bf69ed13038c0d4a80
5f5898bf69ed13038c0d4a80- 18false
- 216false
- 32false
- 4None of thesetrue
- Show AnswerHide Answer
- Workspace
- SingleChoice
Answer : 4. "None of these "
Q: A cuboid has six sides of different colours. The red side is opposite to black, the blue side is adjacent to white. The brown side is adjacent to blue. The red side is face down.. Which one of the following would be the opposite to brown?
880 062ea7006ceafbe581b77050c
62ea7006ceafbe581b77050c- 1Redfalse
- 2Blackfalse
- 3Whitetrue
- 4Bluefalse
- Show AnswerHide Answer
- Workspace
- SingleChoice
Answer : 3. "White"
Q: Position of Dices are given below:
Identify the number when top is 5 what will be at bottom?
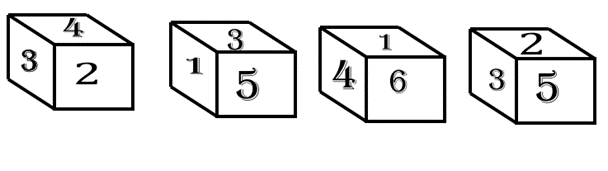
1730 16257c6580560954965a64004
6257c6580560954965a64004- 16false
- 23false
- 34true
- 42false
- Show AnswerHide Answer
- Workspace
- SingleChoice
Answer : 3. "4"
Q: A cuboid whose length is 6 cm. width 4 cm. and height 1 cm. Is.Two faces measuring 4 cm. x1 cm. It is painted black. Two fruits measuring 6 cm. x1 cm. is painted red and is 6 cm. x4 cm. The two faces are painted green. This cuboid is divided by 1 cm. x1 cm. x1 cm. How many cubes have green color on two faces and no color on the remaining four faces -
1474 0619b91efc1c85f0fbf3b2508
619b91efc1c85f0fbf3b2508- 112false
- 28true
- 310false
- 44false
- Show AnswerHide Answer
- Workspace
- SingleChoice
Answer : 2. "8"
Q: A cuboid whose length is 6 cm. width 4 cm. and height 1 cm. Is. Two faces measuring 4 cm. x 1 cm. It is painted black. Two faces measuring 6 cm. x 1 cm. is painted red and is 6 cm. x 4 cm. The two faces are painted green. This cuboid is divided by 1 cm. x1 cm. x1 cm. is cut into cubes, then if the faces containing black and green are removed, then how many cubes will remain -
1349 0619b8d6a925df30febe30861
619b8d6a925df30febe30861- 18false
- 24false
- 316true
- 412false
- Show AnswerHide Answer
- Workspace
- SingleChoice
Answer : 3. "16"
Q: A solid cube is made using 64 small cubes. How many of these small cubes are not visible at all -
2019 0619b9053925df30febe30dcd
619b9053925df30febe30dcd- 16false
- 24false
- 32false
- 48true
- Show AnswerHide Answer
- Workspace
- SingleChoice
Answer : 4. "8"
Q: When two dice are thrown simultaneously, what is the probability that the sum of the two numbers that turn up is less than 12? 1813 05b5cc5ffe4d2b4197774b427
5b5cc5ffe4d2b4197774b427- 135/36true
- 217/36false
- 315/36false
- 41/36false
- Show AnswerHide Answer
- Workspace
- SingleChoice
Answer : 1. "35/36"
Explanation :
Answer: A) 35/36 Explanation: When two dice are thrown simultaneously, the probability is n(S) = 6x6 = 36 Required, the sum of the two numbers that turn up is less than 12 That can be done as n(E) = { (1,1), (1,2), (1,3), (1,4), (1,5), (1,6)(2,1), (2,2), (2,3), (2,4), (2,5), (2,6)(3,1), (3,2), (3,3), (3,4), (3,5), (3,6)(4,1), (4,2), (4,3), (4,4), (4,5), (4,6)(5,1), (5,2), (5,3), (5,4), (5,5), (5,6)(6,1), (6,2), (6,3), (6,4), (6,5) } = 35 Hence, required probability = n(E)/n(S) = 35/36.
Q: In a big cube, there are only two faces colored total cubes are 24. Find out how many small cubes are there in this big cube?
1650 060be0129943add67dd72fae1
60be0129943add67dd72fae1- 164true
- 224false
- 3125false
- 4343false
- Show AnswerHide Answer
- Workspace
- SingleChoice